Line bet, one of the following three cases will happen: Case 1: If the come out roll yields a sum of 7 or 11, then. The player makes a $90 profit. Case 2: If the come out roll yields a sum of 2, 3 or 12, then. The player loses his /her $90 bet. Case 3: If a point (a sum of 4, 5, 6, 8, 9, or 10) is rolled on. The essential starting ground for craps odds and probabilities is with the dice roll itself. Since bets are made based on the potential outcome of the dice, knowing how often, or how infrequently a number combination will occur is the foundation for betting odds in craps. Craps is undoubtedly the most popular dice game of all time and a favorite of millions of players across the world. The game is based solely on chance but players should not be tricked by its apparent simplicity. Contrary to popular belief, craps is not only about rolling the dice.

Introduction

Before you play any dice game it is good to know the probability of any given total to be thrown. First lets look at the possibilities of the total of two dice. The table below shows the six possibilities for die 1 along the left column and the six possibilities for die 2 along the top column. The body of the table shows the sum of die 1 and die 2.

Craps - Wizard Of Odds - Wizard Of Odds

Two Dice Totals

Die 1	Die 2
	1	2	3	4	5	6
1	2	3	4	5	6	7
2	3	4	5	6	7	8
3	4	5	6	7	8	9
4	5	6	7	8	9	10
5	6	7	8	9	10	11
6	7	8	9	10	11	12

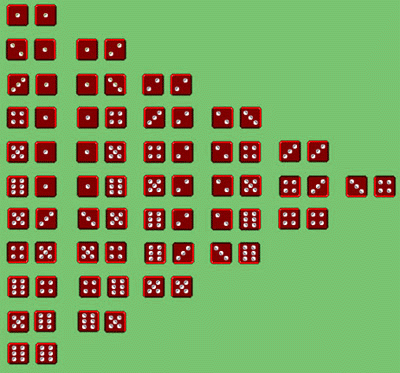
The colors of the body of the table illustrate the number of ways to throw each total. The probability of throwing any given total is the number of ways to throw that total divided by the total number of combinations (36). In the following table the specific number of ways to throw each total and the probability of throwing that total is shown.

Total	Number of Combinations	Probability
2	1	2.78%
3	2	5.56%
4	3	8.33%
5	4	11.11%
6	5	13.89%
7	6	16.67%
8	5	13.89%
9	4	11.11%
10	3	8.33%
11	2	5.56%
12	1	2.78%
Total	36	100%

The following shows the probability of throwing each total in a chart format. As the chart shows the closer the total is to 7 the greater is the probability of it being thrown.

The Field Bet Example

Now that we understand the probability of throwing each total we can apply this information to the dice games in the casinos to calculate the house edge. For example consider the field bet in craps. This bet pays 1:1 (even money) if the next throw is a 3, 4, 9, 10, or 11, 2:1 (double the bet) on the 2, and 3:1 (triple the bet) on the 12. Note that there are 7 totals that win and only 4 that lose which might cause someone who didn't know better to think it was a good gamble.

The player's return can be defined as the sum of the products of the probability of each event and the net return of that event. The following table shows each possible total, the net return, the probability of throwing that total, and the average return. The average return is the product of the net return and the probability. The player's return is the sum of the average returns.

Using Probability To Calculate The Odds In The Game Of Craps ...

Total	Net Return	Probability	Average Return
2	2	0.0278	0.0556
3	1	0.0556	0.0556
4	1	0.0833	0.0833
5	-1	0.1111	-0.1111
6	-1	0.1389	-0.1389
7	-1	0.1667	-0.1667
8	-1	0.1389	-0.1389
9	1	0.1111	0.1111
10	1	0.0833	0.0833
11	1	0.0556	0.0556
12	3	0.0278	0.0834
Total	1	-0.0278

The last row shows the player's return to be -.0278, in other words for every $1 bet the player can expect to lose 2.78 cents. The player's loss is the house's gain so the house edge is the product of -1 and the player's return, in this case 0.0278 or 2.78%.

For the probabilities in the sum of more than two dice please see my probabilities for 1 to 25 dice section.

Craps -- From Wolfram MathWorld

Written by: Michael Shackleford